 |
|
|||
|
Business Card Menger Sponge Exhibit - Introduction
|
FRACTAL
FRAGMENTS |
|||
| In the late nineteenth
century, mathematicians began to explore a kind of form they had hitherto
not been aware of. To all at the time these aberant seemed “monstrous”
for they did not fit the classical pattern of Euclid and Newton. Regarded
at first as pathological, they were mathematical kin to the cubist
paintings and atonal music that were soon upsetting established standards
in the arts. In 1975 Benoit Mandelbrot named the “fractals.”
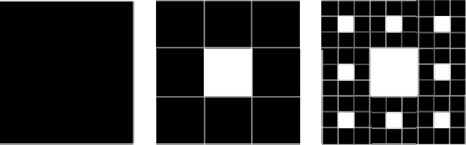
Despite their complexity, many fractals are simple to describe and
rules that generate them are often trivial to state. Fractals arise from the application of iterative rules: Do something once to a primitive form, such as the square below, then repeat the process on successively smaller scales … ad infinitum. |
||||
 |
||||
The resulting
forms often exhibit an enigmatic ambiguity, hovering between two
adjacent dimensions. Taken to its infinite conclusion the Sierpinski
Carpet pictured here dissolves into a foam whose final structure
has no area whatever yet possesses a perimeter that is infinitely
long. Like the skeleton of a beast whose flesh has vanished, the
concluding form is without substance – it occupies a planar
surface, but no longer fills it. |
||||
 |
||||
| In the1920’s
a young Austrian named Karl Menger extended the work begun by his
mathematical predecessor Sierpinski. Menger attended a course of
lectures by Professor Hans Hahn at the University of Vienna entitled
What’s New Concerning the Concept of the Curve; under
Hahn’s encouragement he embarked on an exploration of the
concept of dimension that him to an expanded definition of this
seemingly obvious term. Several years later Menger reported his
discovery of a three-dimensional version of Sierpinski’s Carpet,
which came to be known as the Menger Sponge. Where the Carpet is
poised between a line and a plane, the Sponge hovers of the boundary
of the plane and the solid - its fractional dimension is 2.73. |
||||
 |
||||
In 1995, Dr Jeannine Mosely set out to construct a level three Menger Sponge from business cards. After 9 years of effort involving hundreds of folders all over America, the Business Card Sponge was completed. In August/September 2006 the Institute For Figuring curated an exhibition of the Business Card Sponge at Machine Project [Link] in Los Angeles. Dr. Jeannine Mosely is one of the pioneers in the emerging field of computational origami, a branch of mathematics that explores the formal properties and potentialities of folded paper. An expert on the subfields of business card origami and minimalist origami (in which the practitioner is limited to only four folds), she also conducts research on curved crease origami, investigating the forms that can result from non-linear foldings. Dr Moseley was trained as an electrical engineer at MIT and works in the computer graphics industry writing three-dimensional modeling software. |
||||
| [ next ] | ||||
| © 2003–2018 The Institute For Figuring | ||||