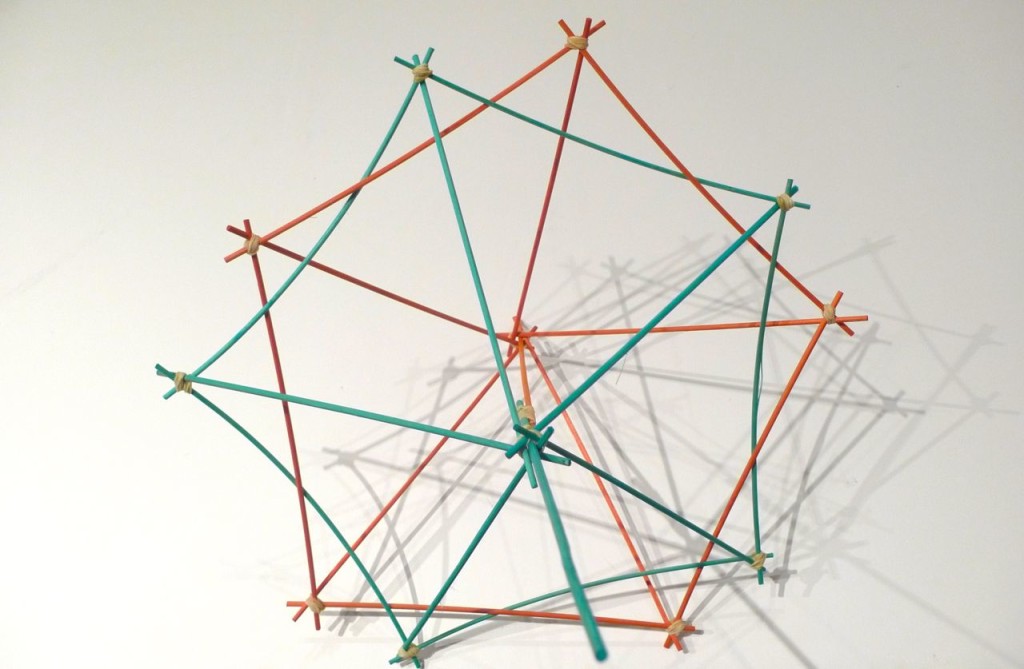
There are lots of ways to connect sticks. A critical issue is the number of sticks that meet at each vertex. The form seen here is derived from the icosahedron, a Platonic solid that has five edges connecting at every vertex giving them pentagonal symmetry. Here, two pentagons – one orange and one blue – are woven together at the mid-plane, then each color is connected by a cone of the same-colored struts which all meet at a vertex. The resulting form is composed of two “stellated pentagons” offset at a 36 degree angle from one another. At our workshop today, the IFF’s Christina Simons realized that by putting a stick through the center the form could be turned into a charming spinning toy. It’s shadow reveals elegant symmetries hidden within the structure.
–Margaret Wertheim, Institute For Figuring